JEE Main Previous Year Question of Math with Solutions are available at eSaral. Practicing JEE Main Previous Year Papers Questions of mathematics will help the JEE aspirants in realizing the question pattern as well as help in analyzing weak & strong areas.
eSaral helps the students in clearing and understanding each topic in a better way. eSaral is providing complete chapter-wise notes of Class 11th and 12th both for all subjects.
Besides this, eSaral also offers NCERT Solutions, Previous year questions for JEE Main and Advance, Practice questions, Test Series for JEE Main, JEE Advanced and NEET, Important questions of Physics, Chemistry, Math, and Biology and many more.
Download eSaral app for free study material and video tutorials.
Q. If $\overrightarrow{\mathrm{u}}, \overrightarrow{\mathrm{v}}, \overrightarrow{\mathrm{w}}$ are non-coplanar vectors and $\mathrm{p}, \mathrm{q}$ are real numbers, then the equality $[3 \vec{u} p \vec{v} p \vec{w}]-[p \vec{v} \vec{w} q \vec{u}]-[2 \vec{w} q \vec{v} q \vec{u}]=0$ holds for $:-$
(1) More than two but not all values of (p,q)
(2) All values of (p, q)
(3) Exactly one value of (p, q)
(4) Exactly two values of (p, q)
[AIEEE-2009]
Ans. (3)
Q. Let $\vec{a}=\hat{j}-\hat{k}$ and $\vec{c}=\hat{i}-\hat{j}-\hat{k} .$ Then the vector $\vec{b}$ satisfying $\vec{a} \times \vec{b}+\vec{c}=\overrightarrow{0}$ and $\vec{a} . \vec{b}=3$ is :
(1) $-\hat{\mathrm{i}}+\hat{\mathrm{j}}-2 \hat{\mathrm{k}}$
(2) $2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+2 \hat{\mathrm{k}}$
(3) $\hat{\mathrm{i}}-\hat{\mathrm{j}}-2 \hat{\mathrm{k}}$
(4) $\hat{\mathrm{i}}+\hat{\mathrm{j}}-2 \hat{\mathrm{k}}$
[AIEEE-2010]
Ans. (1)
$(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}})+\overrightarrow{\mathrm{c}}=0$
$(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}})=-\overrightarrow{\mathrm{c}}$
$\Rightarrow \overrightarrow{\mathrm{a}} \times(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}})=-\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{c}} \Rightarrow(\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}) \overrightarrow{\mathrm{a}}-|\overrightarrow{\mathrm{a}}|^{2} \overrightarrow{\mathrm{b}}=-\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{c}}$
$\Rightarrow 3(\overrightarrow{\mathrm{j}}-\overrightarrow{\mathrm{k}})-2 \overrightarrow{\mathrm{b}}=-(-2 \mathrm{i}-\mathrm{j}-\mathrm{k})$
\[(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{c}}=-2 \mathrm{i}-\mathrm{j}-\mathrm{k})\]
$\Rightarrow 2 \overrightarrow{\mathrm{b}}=(-2 \mathrm{i}+2 \mathrm{j}-4 \mathrm{k}) \quad \Rightarrow \overrightarrow{\mathrm{b}}=-\mathrm{i}+\mathrm{j}-2 \mathrm{k}$
Q. The vectors $\overrightarrow{\mathrm{a}}$ and $\overrightarrow{\mathrm{b}}$ are not perpendicular and $\overrightarrow{\mathrm{c}}$ and $\overrightarrow{\mathrm{d}}$ are two vectors satisfying: $\overrightarrow{\mathrm{b}} \times \overrightarrow{\mathrm{c}}=\overrightarrow{\mathrm{b}} \times \overrightarrow{\mathrm{d}}$ and $\overrightarrow{\mathrm{a} .} \overrightarrow{\mathrm{d}}=0 .$ Then the vector $\overrightarrow{\mathrm{d}}$ is equal to :-
$(1) \overrightarrow{\mathrm{b}}+\left(\frac{\overrightarrow{\mathrm{b}} \cdot \overrightarrow{\mathrm{c}}}{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}}\right) \overrightarrow{\mathrm{c}}$
(2) $\overrightarrow{\mathrm{c}}-\left(\frac{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}}{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}}\right) \overrightarrow{\mathrm{b}}$
(3) $\overrightarrow{\mathrm{b}}-\left(\frac{\overrightarrow{\mathrm{b}} \cdot \overrightarrow{\mathrm{c}}}{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}}\right) \overrightarrow{\mathrm{c}}$
(4) $\overrightarrow{\mathrm{c}}+\left(\frac{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}}{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}}\right) \overrightarrow{\mathrm{b}}$
[AIEEE-2011]
Ans. (2)
Q. If $\overrightarrow{\mathrm{a}}=\frac{1}{\sqrt{10}}(3 \hat{\mathrm{i}}+\hat{\mathrm{k}})$ and $\overrightarrow{\mathrm{b}}=\frac{1}{7}(2 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}-6 \hat{\mathrm{k}}),$ then the value of $(2 \overrightarrow{\mathrm{a}}-\overrightarrow{\mathrm{b}}) \cdot[(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}) \times(\overrightarrow{\mathrm{a}}+2 \overrightarrow{\mathrm{b}})]$ is 1:
(1) 5 (2) 3 (3) – 5 (4) – 3
[AIEEE-2011]
Ans. (3)
$=-4 a^{2}-b^{2}+4 \vec{a} \cdot \vec{b}=-5$
$=-4 a^{2}-b^{2}+4 \vec{a} \cdot \vec{b}=-5$
Q. Let $\vec{a}, \vec{b}, \vec{c}$ be three non-zero vectors which are pairwise non-collinear. If $\vec{a}+3 \vec{b}$ is collinear with $\overrightarrow{\mathrm{c}}$ and $\overrightarrow{\mathrm{b}}+2 \overrightarrow{\mathrm{c}}$ is colliner with $\overrightarrow{\mathrm{a}},$ then $\overrightarrow{\mathrm{a}}+3 \overrightarrow{\mathrm{b}}+6 \overrightarrow{\mathrm{c}}$ is:
(1) $\overrightarrow{\mathrm{a}}+\overrightarrow{\mathrm{c}}$ ( 2)$\quad \vec{a}$ ( 3)$\quad \overrightarrow{\mathrm{c}}$ ( 4) $\overrightarrow{0}$
[AIEEE-2011]
Ans. (4)
Q. Let $\hat{\mathbf{a}}$ and $\hat{\mathbf{b}}$ be two unit vectors. If the vectors $\overrightarrow{\mathbf{c}}=\hat{\mathbf{a}}+2 \hat{\mathbf{b}}$ and $\overrightarrow{\mathbf{d}}=5 \hat{\mathbf{a}}-4 \hat{\mathbf{b}}$ are perpendicular to each other, then the angle between $\hat{\mathbf{a}}$ and $\hat{\mathbf{b}}$ is :
(1) $\frac{\pi}{4}$
( 2)$\frac{\pi}{6}$
(3) $\frac{\pi}{2}$
( 4)$\frac{\pi}{3}$
[AIEEE-2012]
Ans. (4)
$\overline{\mathrm{c}} \cdot \overline{\mathrm{d}}=0 \quad \Rightarrow(\hat{\mathrm{a}}+2 \hat{\mathrm{b}}) \cdot(5 \hat{\mathrm{a}}-4 \hat{\mathrm{b}})=0$
$\Rightarrow 5-8+6 \hat{a} \cdot \hat{b}=0$
$\Rightarrow \hat{\mathrm{a}} \cdot \hat{\mathrm{b}}=1 / 2 \Rightarrow \cos \theta=1 / 2$
$\Rightarrow \theta=\frac{\pi}{3}$
Q. Let ABCD be a parallelogram such that $\overrightarrow{\mathrm{AB}}=\overrightarrow{\mathrm{q}}, \overrightarrow{\mathrm{AD}}=\overrightarrow{\mathrm{p}}$ and $\angle \mathrm{BAD}$ be an acute angle. If $\overrightarrow{\mathrm{r}}$ is the vector that coincides with the altitude directed from the vertex $B$ to the side $A D,$ then $\overrightarrow{\mathrm{r}}$ is given by :
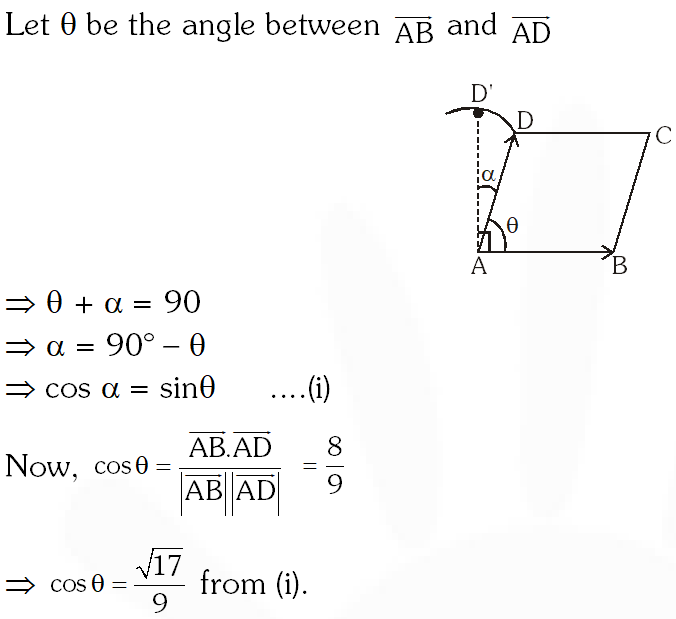[AIEEE-2012]
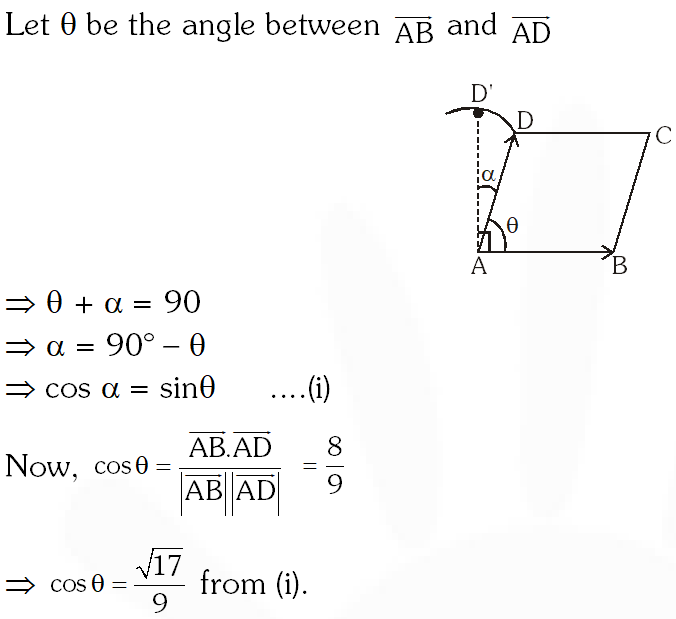[AIEEE-2012]
Ans. (3)
Q. If the vectors $\overrightarrow{\mathrm{AB}}=3 \hat{\mathrm{i}}+4 \hat{\mathrm{k}}$ and $\overrightarrow{\mathrm{AC}}=5 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+4 \hat{\mathrm{k}}$ are the sides of a triangle ABC, then the length of the median through A is :
(1) $\sqrt{18}$
(2) $\sqrt{72}$
(3) $\sqrt{33}$
(4) $\sqrt{45}$
[JEE-MAINS 2013]
Ans. (3)
$\overrightarrow{\mathrm{AD}}=\frac{\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{AC}}}{2}=4 \hat{\mathrm{j}}-\hat{\mathrm{j}}+4 \hat{\mathrm{k}}$
$|\overrightarrow{\mathrm{AD}}|=\sqrt{33}$
$\overrightarrow{\mathrm{AD}}=\frac{\overrightarrow{\mathrm{AB}}+\overrightarrow{\mathrm{AC}}}{2}=4 \hat{\mathrm{j}}-\hat{\mathrm{j}}+4 \hat{\mathrm{k}}$
$|\overrightarrow{\mathrm{AD}}|=\sqrt{33}$
Q. Let $\overrightarrow{\mathrm{a}}=2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+\hat{\mathrm{k}}, \overrightarrow{\mathrm{b}}=\hat{\mathrm{i}}+2 \hat{\mathrm{j}}-\hat{\mathrm{k}}$ and $\overrightarrow{\mathrm{c}}=\hat{\mathrm{i}}+\hat{\mathrm{j}}-2 \hat{\mathrm{k}}$ be three vectors. A vectors. A vectors of the type $\overrightarrow{\mathrm{b}}+\lambda \overrightarrow{\mathrm{c}}$ for some scalar $\lambda,$ whose projection on $\overrightarrow{\mathrm{a}}$ is of magnitude $\sqrt{\frac{2}{3}},$ is :
(1) $2 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}-3 \hat{\mathrm{k}}$
(2) $2 \hat{\mathrm{i}}+\hat{\mathrm{j}}+5 \hat{\mathrm{k}}$
(3) $2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+5 \hat{\mathrm{k}}$
(4) $2 \hat{i}+3 \hat{j}+3 \hat{k}$
[JEE-MAINS Online 2013]
Ans. (1)
$\frac{|(\overline{\mathrm{b}}+\lambda \overline{\mathrm{c}}) \cdot \overline{\mathrm{a}}|}{|\overline{\mathrm{a}}|}=\sqrt{\frac{2}{3}}$
$\left|[(1+\lambda) \hat{\mathrm{i}}+(2+\lambda) \hat{\mathrm{j}}+(-1-2 \lambda) \hat{\mathrm{k}}] \cdot \frac{2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+\hat{\mathrm{k}}}{\sqrt{6}}\right|=\sqrt{\frac{2}{3}}$
$|(2+2 \lambda-2-\lambda-1-2 \lambda)|=2$
$|\lambda+1|=2$
$\lambda+1=\pm 2$
$\lambda=1,-3$
Vect $=2 \hat{\mathrm{i}}+3 \hat{\mathrm{j}}-3 \hat{\mathrm{k}}$ or $-2 \hat{\mathrm{i}}-\hat{\mathrm{j}}+5 \hat{\mathrm{k}}$
Q. Let $\vec{a}=2 \hat{i}+\hat{j}-2 \hat{k}, \vec{b}=\hat{i}+\hat{j} .$ If $\vec{c}$ is a vector such that $\vec{a} \bullet \vec{c}=|\vec{c}|,|\vec{c}-\vec{a}|=2 \sqrt{2}$ and the angle between $\vec{a} \times \vec{b}$ and $\vec{c}$ is $30^{\circ},$ then $|(\vec{a} \times \vec{b}) \times \vec{c}|$ equals :
( 1)$\frac{3}{2}$
(2) 3
( 3)$\frac{1}{2}$
(4) $\frac{3 \sqrt{3}}{2}$
[JEE-MAINS Online 2013]
Ans. (1)
$|\bar{c}|^{2}+|\bar{a}|^{2}-2 \bar{a} \bar{c}=8$
$|\bar{c}|^{2}+9-2|\bar{c}|=8$
$|\overline{\mathrm{c}}|=1$
$|(\overline{\mathrm{a}} \times \overline{\mathrm{b}}) \times \overline{\mathrm{c}}|=|\overline{\mathrm{a}} \times \overline{\mathrm{b}}||\overline{\mathrm{c}}| \sin 30^{\circ}$
$=\frac{|\overline{\mathrm{a}} \times \overline{\mathrm{b}}|}{2}$
$|\overline{\mathrm{a}} \times \overline{\mathrm{b}}|=\left|\begin{array}{ccc}{\hat{\mathrm{i}}} & {\hat{\mathrm{j}}} & {\hat{\mathrm{k}}} \\ {2} & {1} & {-2} \\ {1} & {1} & {0}\end{array}\right|$
$=|2 \hat{\mathbf{i}}+2 \hat{\mathbf{j}}+\hat{\mathbf{k}}|=3$
Q. If $[\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}} \overrightarrow{\mathrm{b}} \times \overrightarrow{\mathrm{c}} \times \overrightarrow{\mathrm{a}}]=\lambda[\overrightarrow{\mathrm{a}} \overrightarrow{\mathrm{b}} \overrightarrow{\mathrm{c}}]^{2}$ then $\lambda$ is equal to :
(1) 2 (2) 3 (3) 0 (4) 1
[JEE(Main)-2014]
Ans. (4)
$[\vec{a} \times \vec{b} \quad \vec{b} \times \vec{c} \quad \vec{c} \times \vec{a}]=(\vec{a} \times \vec{b}) \cdot((\vec{b} \times \vec{c}) \times(\vec{c} \times \vec{a}))$
$=(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}) \cdot((\overrightarrow{\mathrm{b}} \overrightarrow{\mathrm{c}} \cdot \overrightarrow{\mathrm{a}}) \overrightarrow{\mathrm{c}}-[\overrightarrow{\mathrm{b}} \overrightarrow{\mathrm{c}} \cdot \overrightarrow{\mathrm{c}}] \overrightarrow{\mathrm{a}})=[\overrightarrow{\mathrm{a}} \overrightarrow{\mathrm{b}} \overrightarrow{\mathrm{c}}]^{2}$
Q. Let $\vec{a}, \vec{b}$ and $\vec{c}$ be three non-zero vectors such that no two of them are collinear and $(\vec{a}, \times \vec{b}) \times \vec{c}=\frac{1}{3}|\vec{b}||\vec{c}| \vec{a} \cdot$ If $\theta$ is the angle between vectors $\vec{b}$ and $\vec{c},$ then a value of $\sin \theta$ is :
[JEE(Main)-2015]
[JEE(Main)-2015]
Ans. (3)
$(\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}) \overrightarrow{\mathrm{b}}-(\overrightarrow{\mathrm{b}} \cdot \overrightarrow{\mathrm{c}}) \overrightarrow{\mathrm{a}}=\frac{1}{3}|\overrightarrow{\mathrm{b}}||\overrightarrow{\mathrm{c}}| \overrightarrow{\mathrm{a}}$
$\Rightarrow\left[\begin{array}{c}{\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}=0} \\ {-\overrightarrow{\mathrm{b}} \cdot \overrightarrow{\mathrm{c}}=\frac{1}{3}|\overrightarrow{\mathrm{b}} \| \overrightarrow{\mathrm{c}}|}\end{array}\right.$
$\Rightarrow \cos \theta=-\frac{1}{3} \Rightarrow \sin \theta=\frac{2 \sqrt{2}}{3}$
Q. Let $\overrightarrow{\mathrm{a}}, \overrightarrow{\mathrm{b}}$ and $\overrightarrow{\mathrm{c}}$ be three unit vectors such that $\overrightarrow{\mathrm{a}} \times(\overrightarrow{\mathrm{b}} \times \overrightarrow{\mathrm{c}})=\frac{\sqrt{3}}{2}(\overrightarrow{\mathrm{b}}+\overrightarrow{\mathrm{c}}) .$ If $\overrightarrow{\mathrm{b}}$ is not parallel to $\overrightarrow{\mathrm{c}}$ then the angle between a and $\overrightarrow{\mathrm{b}}$ is :-
(1) $\frac{5 \pi}{6}$ (2) $\frac{3 \pi}{4}$ (3) $\frac{\pi}{2}$ (4) $\frac{2 \pi}{3}$
[JEE(Main)-2016]
Ans. (1)
$\left(\overrightarrow{\mathrm{a} .} \overrightarrow{\mathrm{c}}-\frac{\sqrt{3}}{2}\right) \overrightarrow{\mathrm{b}}-\left(\overrightarrow{\mathrm{a} .} \overrightarrow{\mathrm{b}}+\frac{\sqrt{3}}{2}\right) \overrightarrow{\mathrm{c}}=0$
$\Rightarrow \overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}=\cos \theta=-\sqrt{3} / 2 \Rightarrow \theta=5 \pi / 6$
Q. Let $\overrightarrow{\mathrm{a}}=2 \hat{\mathrm{i}}+\hat{\mathrm{j}}-2 \hat{\mathrm{k}}$ and $\overrightarrow{\mathrm{b}}=\hat{\mathrm{i}}+\hat{\mathrm{j}} .$ Let $\overrightarrow{\mathrm{c}}$ be a vector such that $|\overrightarrow{\mathrm{c}}-\overrightarrow{\mathrm{a}}|=3,|(\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}) \times \overrightarrow{\mathrm{c}}|=3$ and the angle between $\overrightarrow{\mathrm{c}}$ and $\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}$ be $30^{\circ} .$ Then $\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}$ is equal to :
( 1)$\frac{1}{8}$ (2) $\frac{25}{8}$ (3) 2 (4) 5
[JEE(Main)-2018]
Ans. (3)
$\overrightarrow{\mathrm{a}}=2 \hat{\mathrm{i}}+\hat{\mathrm{j}}-2 \hat{\mathrm{k}}, \quad \overrightarrow{\mathrm{b}}=\hat{\mathrm{i}}+\hat{\mathrm{j}}$ and $|\overrightarrow{\mathrm{a}}|=3$
$\therefore \overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}=2 \hat{\mathrm{i}}-2 \hat{\mathrm{j}}+\hat{\mathrm{k}}$
$|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}|=3$
Now $:(\vec{a} \times \vec{b}) \times \vec{c}=|\vec{a} \times \vec{b}||\vec{c}| \sin 30 \hat{n}$
$|(\vec{a} \times \vec{b}) \times \vec{c}|=3 \cdot|\vec{c}| \cdot \frac{1}{2}$
$3=3|\overrightarrow{\mathrm{c}}| \cdot \frac{1}{2}$
$\therefore|\overrightarrow{\mathrm{c}}|=2$
Now $:|\overrightarrow{\mathrm{c}}-\overrightarrow{\mathrm{a}}|=3$
$c^{2}+a^{2}-2 \vec{c} \cdot \vec{a}=9$
$4+9-2 \overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}=9$
$\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{c}}=2$
Q. If the position vectors of the vertices $\mathrm{A}, \mathrm{B}$ and $\mathrm{C}$ of a $\Delta \mathrm{ABC}$ are respectively $4 \hat{i}+7 \hat{j}+8 \hat{k}, 2 \hat{i}+3 \hat{j}+4 \hat{k}$ and $2 \hat{i}+5 \hat{j}+7 \hat{k},$ then the position vector of the point, where the bisector of $\angle \mathrm{A}$ meets $\mathrm{BC}$ is :
(1) $\frac{1}{2}(4 \hat{i}+8 \hat{j}+11 \hat{k})$
(2) $\frac{1}{3}(6 \hat{i}+13 \hat{j}+18 \hat{k})$
(3) $\frac{1}{4}(8 \hat{\mathrm{i}}+14 \hat{\mathrm{j}}+19 \hat{\mathrm{k}})$
(4) $\frac{1}{3}(6 \hat{i}+11 \hat{j}+15 \hat{k})$
[JEE(Main)-2018]
Ans. (2)
Q. If $\overrightarrow{\mathrm{a}}, \overrightarrow{\mathrm{b}},$ and $\overrightarrow{\mathrm{c}}$ are unit vectors such that $\overrightarrow{\mathrm{a}}+2 \overrightarrow{\mathrm{b}}+2 \overrightarrow{\mathrm{c}}=\overrightarrow{0},$ then $|\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{c}}|$ is equal to :
[JEE(Main)-2018]
[JEE(Main)-2018]
Ans. (4)
Q. If the angle between the lines, $\frac{x}{2}=\frac{y}{2}=\frac{z}{1}$ and $\frac{5-x}{-2}=\frac{7 y-14}{p}=\frac{z-3}{4}$ is $\cos ^{-1}\left(\frac{2}{3}\right),$ then $p$ is equal to:
[JEE(Main)-2018]
[JEE(Main)-2018]
Ans. (2)
Q. Let $\overrightarrow{\mathrm{a}}=\hat{\mathrm{i}}+\hat{\mathrm{j}}+\hat{\mathrm{k}}, \overrightarrow{\mathrm{c}}=\hat{\mathrm{j}}-\hat{\mathrm{k}}$ and a vector $\overrightarrow{\mathrm{b}}$ be such that $\overrightarrow{\mathrm{a}} \times \overrightarrow{\mathrm{b}}=\overrightarrow{\mathrm{c}}$ and $\overrightarrow{\mathrm{a}} \cdot \overrightarrow{\mathrm{b}}=3 .$ Then $|\overrightarrow{\mathrm{b}}|$ equals :
(1) $\sqrt{\frac{11}{3}}$
(2) $\frac{11}{\sqrt{3}}$
(2) $\frac{11}{\sqrt{3}}$
( 4)$\frac{11}{3}$
[JEE(Main)-2018]
Ans. (1)
Q. Let $\vec{u}$ be a vector coplanar with the vectors $\vec{a}=2 \hat{i}+3 \hat{j}-\hat{k}$ and $\vec{b}=\hat{j}+\hat{k} .$ If $\vec{u}$ is perpendicular to $\overrightarrow{\mathrm{a}}$ and $\overrightarrow{\mathrm{u} . \overrightarrow{\mathrm{b}}}=24,$ then $|\overrightarrow{\mathrm{u}}|^{2}$ is equal to-
(1) 315 (2) 256 (3) 84 (4) 336
[JEE(Main)-2018]
Ans. (4)