What is the angle of elevation of the Sun when the

Let  be the angle of elevation of sun is.

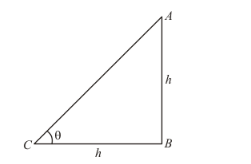
Given that: Height of pole is  meters and length of shadow is  meters. Because length of shadow is equal to the height of pole.

Here we have to find angle of elevation of sun.

So we use trigonometric ratios.

In a triangle,

$\Rightarrow \tan \theta=\frac{A B}{B C}$

$\Rightarrow \tan \theta=\frac{h}{h} \quad\left[\because \tan 45^{\circ}=1\right]$

$\Rightarrow \tan \theta=1$

$\Rightarrow \theta=45^{\circ}$

Hence the angle of elevation of sun is $45^{\circ}$.