Two identical cylindrical vessels are kept on the ground and each contain the same liquid of density d.
Two identical cylindrical vessels are kept on the ground and each contain the same liquid of density $d$. The area of the base of both vessels is $S$ but the height of liquid in one vessel is $x_{1}$ and in the other, $x_{2}$. When both cylinders are connected through a pipe of negligible volume very close to the bottom, the liquid flows from one vessel to the other until it comes to equilibrium at a new height. The change in energy of the system in the process is :
Correct Option: , 4
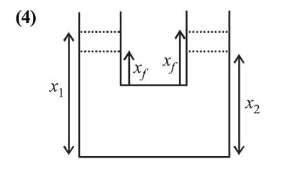
Initial potential energy,
$U_{1}=\left(\rho S x_{1}\right) g \cdot \frac{x_{1}}{2}+\left(\rho S x_{2}\right) g \cdot \frac{x_{2}}{2}$
Final potential energy,
$U_{f}=\left(\rho S x_{f}\right) g \cdot \frac{x_{f}}{2} \times 2$
By volume conservation,
$S x_{1}+S x_{2}=S\left(2 x_{f}\right)$
$x_{f}=\frac{x_{1}+x_{2}}{2}$
When valve is opened loss in potentail energy occur till water level become same.
$\Delta U=U_{i}-U_{f}$
$\Delta U=\rho S g\left[\left(\frac{x_{1}^{2}}{2}+\frac{x_{2}^{2}}{2}\right)-x_{f}^{2}\right]$
$=\rho S g\left[\frac{x_{1}^{2}}{2}+\frac{x_{2}^{2}}{2}-\left(\frac{x_{1}+x_{2}}{2}\right)^{2}\right]$
$=\frac{\rho S g}{2}\left[\frac{x_{1}^{2}}{2}+\frac{x_{2}^{2}}{2}-x_{1} x_{2}\right]=\frac{\rho S g}{4}\left(x_{1}-x_{2}\right)^{2}$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.