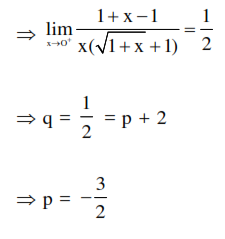Question:
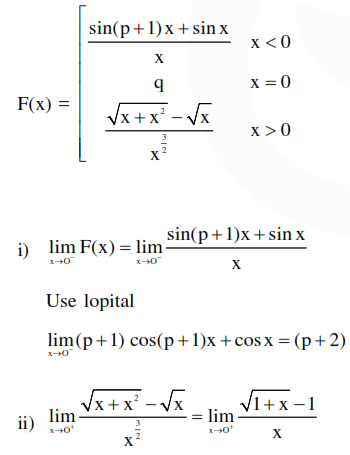
The values of $p$ and $q$ for which the function $f(x)=$ $f(x)= \begin{cases}\frac{\sin (p+1) x+\sin x}{x}, & x<0 \\ q & , x=0 \text { is continuous for } \\ \frac{\sqrt{x+x^{2}}-\sqrt{x}}{x^{\frac{3}{2}}}, & x>0\end{cases}$ all $x$ in $R$, are :-
Correct Option: 1
Solution: