The results given in the below table were obtained during kinetic studies of the following reaction :
The results given in the below table were obtained during kinetic studies of the following reaction :
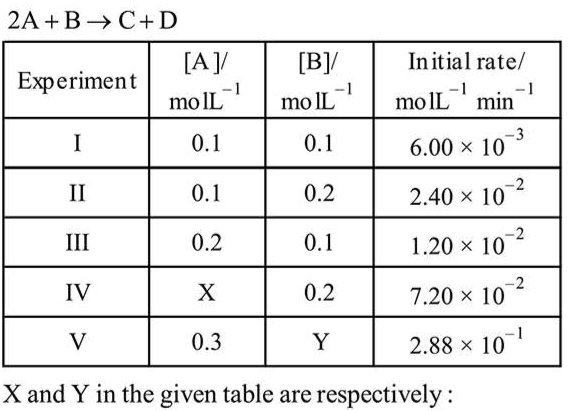
Correct Option: , 3
$\operatorname{Rate}(\mathrm{R})=\mathrm{k}[\mathrm{A}]^{\mathrm{a}}[\mathrm{B}]^{\mathrm{b}}$
$\operatorname{Exp} \mathrm{I} \Rightarrow 6.0 \times 10^{-3}=\mathrm{k}[0.1]^{\mathrm{a}}[0.1]^{\mathrm{b}}$
$\operatorname{Exp} \mathrm{II} \Rightarrow 24.0 \times 10^{-3}=\mathrm{k}[0.1]^{\mathrm{a}}[0.2]^{\mathrm{b}}$
$\operatorname{Exp} \mathrm{III} \Rightarrow 12.0 \times 10^{-3}=\mathrm{k}[0.2]^{\mathrm{a}}[0.1]^{\mathrm{b}}$
$\operatorname{Exp} \mathrm{IV} \Rightarrow 72 \times 10^{-3}=\mathrm{k}[\mathrm{X}]^{\mathrm{a}}[0.2]^{\mathrm{b}}$
$\operatorname{Exp} \mathrm{V} \Rightarrow 288 \times 10^{-3}=\mathrm{k}[0.3]^{\mathrm{a}}[\mathrm{Y}]^{\mathrm{b}}$
From Exp I \& II,
$\Rightarrow \frac{6.0 \times 10^{-3}}{24 \times 10^{-3}}=\frac{\mathrm{k}[0.1]^{\mathrm{a}}[0.1]^{\mathrm{b}}}{\mathrm{k}[0.1]^{\mathrm{a}}[0.2]^{\mathrm{b}}}$
$\Rightarrow \frac{1}{4}=\left(\frac{0.1}{0.2}\right)^{\mathrm{b}} \Rightarrow\left(\frac{1}{2}\right)^{2}=\left(\frac{1}{2}\right)^{\mathrm{b}}$
$\therefore \mathrm{b}=2$
Similarly, from $\exp$ I \& III we get
$\frac{1}{2}=\left(\frac{1}{2}\right)^{\mathrm{a}} \Rightarrow \mathrm{a}=1$
From Exp. II \& IV,
$\Rightarrow \frac{24 \times 10^{-3}}{72 \times 10^{-3}}=\frac{\mathrm{k}[0.1]^{\mathrm{a}}[0.2]^{\mathrm{b}}}{\mathrm{k}[\mathrm{X}]^{\mathrm{a}}[0.2]^{\mathrm{b}}}$
$\Rightarrow \frac{1}{3}=\left(\frac{0.1}{\mathrm{X}}\right)^{\mathrm{a}} \Rightarrow \frac{1}{3}=\left(\frac{0.1}{\mathrm{X}}\right)^{1}$
$\therefore \mathrm{X}=0.3$
From Exp. I \&V,
$\Rightarrow \frac{6.0 \times 10^{-3}}{288 \times 10^{-3}}=\left(\frac{0.1}{0.3}\right)^{\mathrm{a}}\left(\frac{0.1}{\mathrm{Y}}\right)^{\mathrm{b}}$
$\Rightarrow \frac{1}{48}=\left(\frac{1}{3}\right)^{1}\left(\frac{0.1}{Y}\right)^{2} \Rightarrow \frac{3}{48}=\left(\frac{0.1}{Y}\right)^{2} \Rightarrow \frac{1}{16}=\left(\frac{0.1}{Y}\right)^{2}$
$\Rightarrow\left(\frac{1}{4}\right)^{2}=\left(\frac{0.1}{Y}\right)^{2} \Rightarrow \frac{1}{4}=\frac{0.1}{Y}$
$\therefore \mathrm{Y}=0.4$
