Question:
The random variable X can take only the values 0, 1, 2. Given that P(X = 0) = P (X = 1) = p and that E(X2) = E[X], find the value of p.
Solution:
Given, X = 0, 1, 2 and P(X = 0) = P (X = 1) = p
Let P(X) at X = 2 is x
⇒ p + p + x = 1 ⇒ x = 1 – 2p
Now, we have the following distribution:
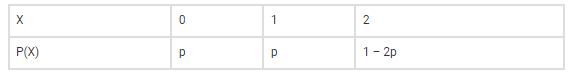
So,
E(X) = 0.p + 1.p + 2(1 – 2p) = p + 2 – 4p = 2 – 3p
And, E(X2) = 0.p + 1.p + 4(1 – 2p) = p + 4 – 8p = 4 – 7p
Also, given E(X) = E(X2)
2 – 3p = 4 – 7p
4p = 2
p = ½
Therefore, the required value of p is ½.
