Question:
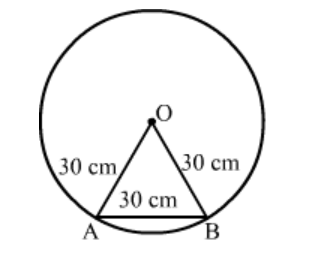
The radius of a circle is 30 cm. Find the length of an arc of this circle, if the length of the chord of the arc is 30 cm.
Solution:
Let AB be the chord and O be the centre of the circle.
Here,
AO = BO = AB = 30 cm
Therefore, $\Delta A O B$ is an equilateral triangle.
Now,
Radius = 30 cm
$\theta=60^{\circ}=\left(60 \times \frac{\pi}{180}\right)=\frac{\pi}{3} \operatorname{radian}$
$\theta=\frac{\text { Arc }}{\text { Radius }}$
$\Rightarrow \frac{\pi}{3}=\frac{\operatorname{Arc}}{30}$
$\Rightarrow \operatorname{Arc}=\frac{30 \pi}{3}=10 \pi \mathrm{cm}$