Question:
(a) $3 \pi \mathrm{r}^{2}$
(b) $\frac{\pi r^{3}}{3}$
(C) $\frac{\pi r^{2}}{3}$
(d) $3 \pi r^{3}$
Solution:
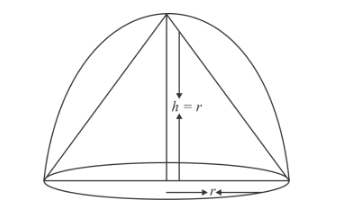
Radius of hemisphere = r
Therefore,
The radius of cone = r
and height h = r
Then,
Volume of cone
$=\frac{1}{3} \pi r^{2} h$
$=\frac{1}{3} \pi r^{2} \times r$
$=\frac{1}{3} \pi r^{3}$ (unit) $^{3}$
Hence, the correct answer is choice (b).
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.