Question:
The maximum area (in sq. units) of a rectangle having its base on the $\mathrm{x}$-axis and its other two vertices on the parabola, $y=12-x^{2}$ such that the rectangle lies inside the parabola, is :-
Correct Option: , 3
Solution:
$f(a)=2 a(12-a)^{2}$
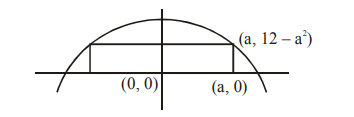
$f^{\prime}(a)=2\left(12-3 a^{2}\right)$
maximum at $\mathrm{a}=2$
maximum area $=\mathrm{f}(2)=32$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.