Question:
The locus of the centres of the circles, which touch the circle, $x^{2}+y^{2}=1$ externally, also touch the $y$-axis and lie in the first quadrant, is:
Correct Option: 2,
Solution:
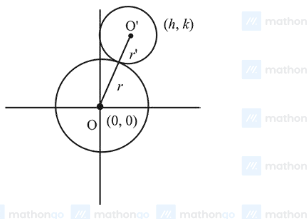
Let centre of required circle is $(h, k)$.
$\therefore \mathrm{OO}^{\prime}=r+r^{\prime} \quad$ [By the diagram]
$\Rightarrow \sqrt{h^{2}+k^{2}}=1+h$
$\Rightarrow h^{2}+k^{2}=1+h^{2}+2 h$
$\Rightarrow k^{2}=1+2 h$
$\therefore$ locus is $y=\sqrt{1+2 x}$
$x \geq 0$