The incident ray, reflected ray and the outward drawn normal are denoted by

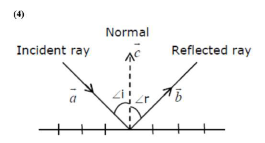
We see from the diagram that because of the law of reflection, the component of the unit vector $\vec{a}$ along $\vec{b}$ changes sign on reflection while the component parallel to the mirror remain unchanges, $\vec{a}=\overrightarrow{a_{11}}+\overrightarrow{a_{1}}$

and $\overrightarrow{a_{\perp}}=\vec{c}(\vec{a} \cdot \vec{c})$

we see that the reflected unit vector is $\vec{b}=\overrightarrow{a_{11}}-\overrightarrow{a_{\perp}} \Rightarrow \vec{a}-2(\vec{a} \cdot \vec{c}) \vec{c}$