The horizontal distance between two towers is 60 metres.

Let $D E$ be the first tower and $A B$ be the second tower.

Now, $A B=90 \mathrm{~m}$ and $A D=60 \mathrm{~m}$ such that $C E=60 \mathrm{~m}$ and $\angle B E C=30^{\circ}$.

Let $D E=h \mathrm{~m}$ such that $A C=h \mathrm{~m}$ and $B C=(90-h) \mathrm{m}$.

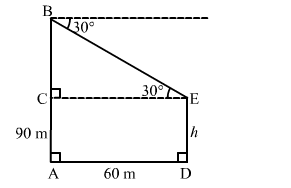
In the right $\Delta B C E$, we have:

$\frac{B C}{C E}=\tan 30^{\circ}=\frac{1}{\sqrt{3}}$

$\Rightarrow \frac{(90-h)}{60}=\frac{1}{\sqrt{3}}$

$\Rightarrow(90-h) \sqrt{3}=60$

$\Rightarrow h \sqrt{3}=90 \sqrt{3}-60$

$\Rightarrow h=90-\frac{60}{\sqrt{3}}=90-34.64=55.36 \mathrm{~m}$

$\therefore$ Height of the first tower $=D E=h=55.36 \mathrm{~m}$