The height of a right circular cone is 20 cm.

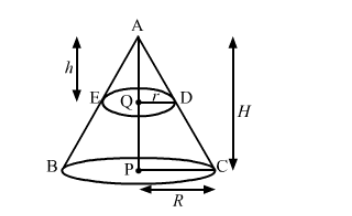
We have,

Height of the given cone, $H=20 \mathrm{~cm}$

Let the radius of the given cone be $R$, the height of the smaller cone be $h$ and the radius of the smaller cone be $r$.

Now, in $\Delta \mathrm{AQD}$ and $\Delta \mathrm{APC}$,

$\angle \mathrm{QAD}=\angle \mathrm{PAC} \quad$ (Common angle)

$\angle \mathrm{AQD}=\angle \mathrm{APC}=90^{\circ}$

So, by AA criteria

$\Delta \mathrm{AQD} \sim \Delta \mathrm{APC}$

$\Rightarrow \frac{\mathrm{AQ}}{\mathrm{AP}}=\frac{\mathrm{QD}}{\mathrm{PC}}$

$\Rightarrow \frac{h}{H}=\frac{r}{R} \quad \ldots$ (i)

Volume of smaller cone $=\frac{1}{8} \times$ Volume of the given cone

$\Rightarrow \frac{\text { Volume of smaller cone }}{\text { Volume of the given cone }}=\frac{1}{8}$

$\Rightarrow \frac{\left(\frac{1}{3} \pi r^{2} h\right)}{\left(\frac{1}{3} \pi R^{2} H\right)}=\frac{1}{8}$

$\Rightarrow\left(\frac{r}{R}\right)^{2} \times\left(\frac{h}{H}\right)=\frac{1}{8}$

$\Rightarrow\left(\frac{h}{H}\right)^{2} \times\left(\frac{h}{H}\right)=\frac{1}{8} \quad[\operatorname{Using}(\mathrm{i})]$

$\Rightarrow\left(\frac{h}{H}\right)^{3}=\frac{1}{8}$

$\Rightarrow \frac{h}{H}=\sqrt[3]{\frac{1}{8}}$

$\Rightarrow \frac{h}{20}=\frac{1}{2}$

$\Rightarrow h=\frac{20}{2}$

$\Rightarrow h=10 \mathrm{~cm}$

$\therefore \mathrm{PQ}=H-h=20-10=10 \mathrm{~cm}$

So, the section is made at the height of 10 cm above the base.