The height of a cone is 60 cm.

It is given that the height of a cone = 60 cm

The volume of a small cone cut on the top by plane parallel to base of given cone
=  volume of given cone

We have to find the height from the base at which section is made.

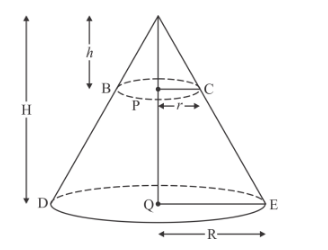
Let R be the radius of base of given cone

Let H be the height of given cone

H = 60 cm

Let r be the radius of base of small cone

Let h be the height of small cone

In ΔAPC and ΔAQE

PC || QE

Therefore

$\angle \mathrm{APC}=\angle \mathrm{AQE} \quad[$ Corresponding angles $]$

$\angle \mathrm{ACP}=\angle \mathrm{AEQ} \quad[$ Corresponding angles $]$

Also $\angle \mathrm{PAC}=\angle \mathrm{QAE}$ [Same angle]

Hence ΔAPC ~ ΔAQE

$\frac{A P}{A Q}=\frac{P C}{Q E}$

$\frac{h}{H}=\frac{r}{R}$.......(1)

Volume of cone $\mathrm{ADE}=\frac{1}{3} \pi R^{2} H$

Volume of cone $\mathrm{ABC}=\frac{1}{3} \pi r^{2} h$

$\frac{\text { Volume of cone } \mathrm{ABC}}{\text { Volume of cone } \mathrm{ADE}}=\frac{\frac{1}{3} \pi r^{2} h}{\frac{1}{3} \pi R^{2} H}$

$\frac{1}{64}=\left(\frac{r}{R}\right)^{2} \times \frac{h}{H}$

$\frac{1}{64}=\left(\frac{h}{H}\right)^{2} \times \frac{h}{H}[$ From $(1)]$

$\frac{1}{64}=\left(\frac{h}{H}\right)^{3}$

$\sqrt[3]{\frac{1}{64}}=\frac{h}{H}$

$\frac{1}{4}=\frac{h}{60}$

$h=15$

The height from the base at which section is made $=H-h$

$=60-15 \mathrm{~cm}$

$=45 \mathrm{~cm}$

Hence Option (c) is correct.