The diagonals of a rhombus are 48 cm and 20 cm long. Find the perimeter of the rhombus.
Diagonals of a rhombus perpendicularly bisect each other. The statement can help us find a side of the rhombus. Consider the following figure.
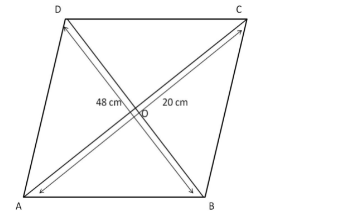
ABCD is the rhombus and AC and BD are the diagonals. The diagonals intersect at point O.
We know:
$\angle D O C=90^{\circ}$
$D O=O B=\frac{1}{2} D B=\frac{1}{2} \times 48=24 \mathrm{~cm}$
Similarly,
$A O=O C=\frac{1}{2} A C=\frac{1}{2} \times 20=10 \mathrm{~cm}$
Using Pythagoras' theorem in the right-angled triangle $\triangle \mathrm{DOC}$, we get:
$D C^{2}=\sqrt{D O^{2}+O C^{2}}$
$=\sqrt{24^{2}+10^{2}}$
$=\sqrt{576+100}$
$=\sqrt{676}$
$=26 \mathrm{~cm}$
DC is a side of the rhombus.
We know that in a rhombus, all sides are equal.
$\therefore$ Perimeter of $A B C D=26 \times 4=104 \mathrm{~cm}$
