The curved surface area of a cylinder is 264 m2

Solution:

The C.S.A. of cylinder

S = 264 m2

The volume of cylinder

V = 924 m3

$2 \pi r h=264$

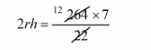....................(i)

$2 r h=84$

$r h=42$

$\pi r^{2} h=924$

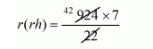...........$. .(i i)$

$r(r h)=42 \times 7$

From eq. (i) and (ii),

We get $r=7$

Putting the value in $(i) \quad h=6$

Hence, $\frac{d}{h}=\frac{14}{6}=\frac{7}{3}$

$d: h=7: 3$

Hence, the correct answer is choice (b).