The conductivity of sodium chloride at 298 K has been determined at different concentrations and the results are given below:
The conductivity of sodium chloride at 298 K has been determined at different concentrations and the results are given below:
Concentration/M 0.001 0.010 0.020 0.050 0.100
102 × κ/S m−1 1.237 11.85 23.15 55.53 106.74
Calculate $\Lambda_{m}$ for all concentrations and draw a plot between $\Lambda_{m}$ and $\mathrm{c}^{1 / 2}$. Find the value of $\Lambda_{m}^{0}$.
Given,
κ = 1.237 × 10−2 S m−1, c = 0.001 M
Then, κ = 1.237 × 10−4 S cm−1, c½ = 0.0316 M1/2
$\therefore \Lambda_{m}=\frac{\kappa}{c}$
$=\frac{1.237 \times 10^{-4} \mathrm{~S} \mathrm{~cm}^{-1}}{0.001 \mathrm{~mol} \mathrm{~L}^{-1}} \times \frac{1000 \mathrm{~cm}^{3}}{\mathrm{~L}}$
= 123.7 S cm2 mol−1
Given,
κ = 11.85 × 10−2 S m−1, c = 0.010M
Then, κ = 11.85 × 10−4 S cm−1, c½ = 0.1 M1/2
$\therefore \Lambda_{m}=\frac{\kappa}{c}$
$=\frac{11.85 \times 10^{-4} \mathrm{~S} \mathrm{~cm}^{-1}}{0.010 \mathrm{~mol} \mathrm{~L}^{-1}} \times \frac{1000 \mathrm{~cm}^{3}}{\mathrm{~L}}$
= 118.5 S cm2 mol−1
Given,
κ = 23.15 × 10−2 S m−1, c = 0.020 M
Then, κ = 23.15 × 10−4 S cm−1, c1/2 = 0.1414 M1/2
$\therefore \Lambda_{m}=\frac{\kappa}{c}$
$=\frac{23.15 \times 10^{-4} \mathrm{~S} \mathrm{~cm}^{-1}}{0.020 \mathrm{~mol} \mathrm{~L}^{-1}} \times \frac{1000 \mathrm{~cm}^{3}}{\mathrm{~L}}$
= 115.8 S cm2 mol−1
Given,
κ = 55.53 × 10−2 S m−1, c = 0.050 M
Then, κ = 55.53 × 10−4 S cm−1, c1/2 = 0.2236 M1/2
$\therefore \kappa=\frac{\kappa}{c}$
$=\frac{55.53 \times 10^{-4} \mathrm{~S} \mathrm{~cm}^{-1}}{0.050 \mathrm{~mol} \mathrm{~L}^{-1}} \times \frac{1000 \mathrm{~cm}^{3}}{\mathrm{~L}}$
= 111.1 1 S cm2 mol−1
Given,
κ = 106.74 × 10−2 S m−1, c = 0.100 M
Then, κ = 106.74 × 10−4 S cm−1, c1/2 = 0.3162 M1/2
$\therefore \Lambda_{m}=\frac{\kappa}{c}$
$=\frac{106.74 \times 10^{-4} \mathrm{~S} \mathrm{~cm}^{-1}}{0.100 \mathrm{~mol} \mathrm{~L}^{-1}} \times \frac{1000 \mathrm{~cm}^{3}}{\mathrm{~L}}$
= 106.74 S cm2 mol−1
Now, we have the following data:
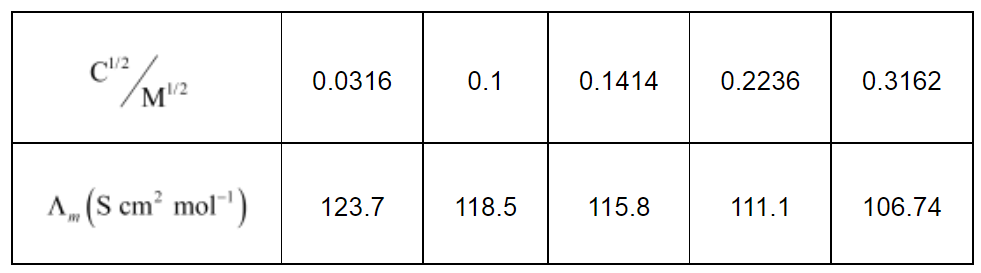
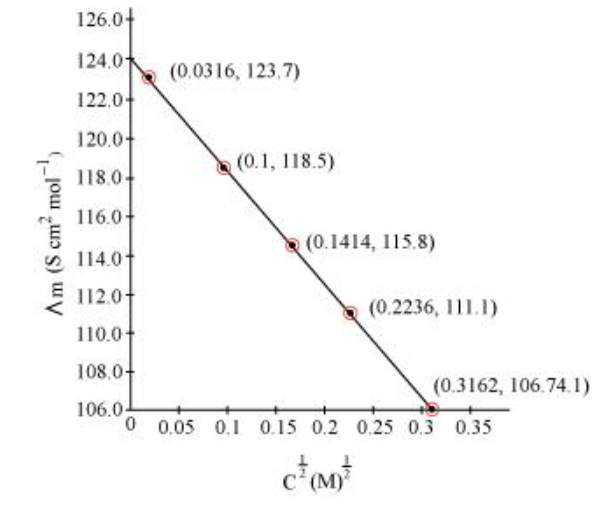
Since the line interrupts $\Lambda_{m}$ at $124.0 \mathrm{~S} \mathrm{~cm}^{2} \mathrm{~mol}^{-1}, \Lambda_{m}^{0}=124.0 \mathrm{~S} \mathrm{~cm}^{2} \mathrm{~mol}^{-1}$.
