Question:
The centre of a wheel rolling on a plane surface moves with a speed $v_{0}$. A particle on the rim of the wheel at the same level as the centre will be moving at a speed $\sqrt{\mathrm{x}} v_{0}$. Then the value of $\mathrm{x}$ is
Solution:
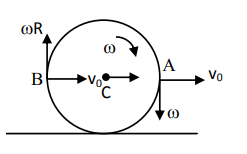
For no slipping $\mathrm{v}_{0}=\omega \mathrm{R}$
$\operatorname{Now} \mathrm{v}_{\mathrm{A}}=\mathrm{v}_{\mathrm{B}}=\sqrt{\mathrm{v}_{0}^{2}+(\omega \mathrm{R})^{2}}$
$=\sqrt{2} \mathrm{v}_{0}$
$\Rightarrow \quad x=2$
