Question:
The area of a triangle with vertices $(-3,0),(3,0)$ and $(0, k)$ is 9 sq. units. The value of $k$ will be
(a) 9
(b) 3
(c) $-9$
(d) 6
Solution:
Given: Area of a triangle with vertices $(-3,0),(3,0)$ and $(0, k)=9$ sq. units
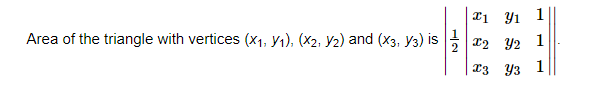
According to the question,
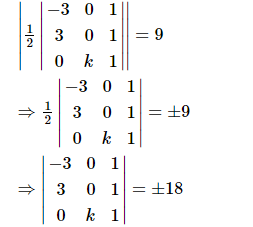
$\Rightarrow\{-3(0-k)-0(3)+1(3 k)\}=\pm 18$
$\Rightarrow\{-3(-k)+1(3 k)\}=\pm 18$
$\Rightarrow\{3 k+3 k\}=\pm 18$
$\Rightarrow 6 k=\pm 18$
$\Rightarrow k=\pm 3$
Hence, the correct option is (b).
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.