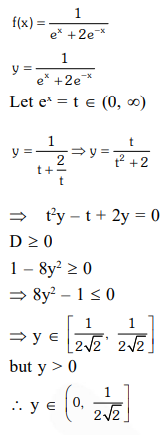Solve this following

Question:

Let $f: R \rightarrow R$ be a continuous function defined by $f(x)=\frac{1}{e^{x}+2 e^{-x}}$

Statement-1 : $\mathrm{f}(\mathrm{c})=\frac{1}{3}$, for some $\mathrm{c} \in \mathrm{R}$

Statement-2 : $0<\mathrm{f}(\mathrm{x}) \leq \frac{1}{2 \sqrt{2}}$, for all $\mathrm{x} \in \mathrm{R}$

 

  1. Statement-1 is true, Statement- 2 is true ; Statement $-2$ is a correct explanation for Statement-1.

  2. Statement-1 is true, Statement $-2$ is true ; Statement $-2$ is not a correct explanation for statement-1.

  3. Statement $-1$ is true, Statement $-2$ is false.

  4. Statement $-1$ is false, Statement $-2$ is true.

Correct Option: 1

Solution:

 

Leave a comment

eSaral Gurukul

NEET

JEE Main

JEE Advanced

Our Telegram Channel

All Study Material