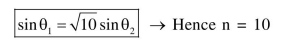
A particle of mass $m$ is moving along the $x$-axis with initial velocity u\hat{i. It collides elastically } with a particle of mass $10 \mathrm{~m}$ at rest and then moves with half its initial kinetic energy (see figure). If $\sin \theta_{1}=\sqrt{\mathrm{n}} \sin \theta_{2}$ then value of $\mathrm{n}$ is


By momentum conservation along $y$ :
$\mathrm{m}_{1} \mathrm{u}_{1} \sin \theta_{1}=\mathrm{m}_{2} \mathrm{u}_{2} \sin \theta_{2}$
i.e. $\quad m u_{1} \sin \theta_{1}=10 m u_{2} \sin \theta_{2}$

$\mathrm{kf}_{\mathrm{m}_{1}}=\frac{1}{2} \mathrm{ki}_{\mathrm{m}_{1}} \quad$ i.e. $\frac{1}{2} \mathrm{mu}_{1}^{2}=\frac{1}{2} \times \frac{1}{2} \mathrm{mu}^{2}$
 .................(ii)
.................(ii)
Also collision is elastic : $\mathrm{k}_{\mathrm{i}}=\mathrm{k}_{\mathrm{f}}$
$\frac{1}{2} \mathrm{mu}^{2}=\frac{1}{2} \mathrm{mu}_{1}^{2}+\frac{1}{2} \cdot 10 \mathrm{~m} \cdot \mathrm{u}_{2}^{2}$
$\frac{1}{2} m u^{2}=\frac{1}{2} \times \frac{1}{2} m u^{2}+\frac{1}{2} \times 10 m \cdot u_{2}^{2}$
$\frac{1}{4} \mathrm{mu}^{2}=\frac{1}{2} \times 10 \times \mathrm{mu}_{2}^{2}$

Putting (ii) \& (iii) in (i)
$\frac{\mathrm{u}}{\sqrt{2}} \sin \theta_{1}=10 \cdot \frac{\mathrm{u}}{\sqrt{20}} \sin \theta_{2}$