An inductance coil has a reactance of $100 \Omega$. When an $\mathrm{AC}$ signal of frequency $1000 \mathrm{~Hz}$ is applied to the coil, the applied voltage leads the current by $45^{\circ}$. The self-inductance of the coil is :
Correct Option: 1

- Reactance of inductance coil
$=\sqrt{\mathrm{R}^{2}+\mathrm{x}_{\mathrm{L}}^{2}}=100$ .............(I)
- $\mathrm{f}=1000 \mathrm{~Hz}$ of applied $\mathrm{AC}$ signal
- Voltage leads current by $45^{\circ}$
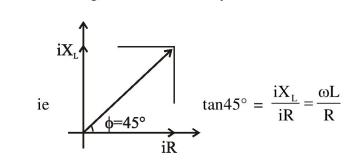
ie $\mathrm{R}=\mathrm{X}_{\mathrm{L}}=\omega \mathrm{L}$
Putting in eqn (i) : $\sqrt{X_{L}^{2}+X_{L}^{2}}=100$
$\sqrt{2} X_{L}=100 \Rightarrow X_{L}=50 \sqrt{2}$
ie $\omega L=50 \sqrt{2}$
$\mathrm{L}=\frac{50 \sqrt{2}}{\omega}=\frac{50 \sqrt{2}}{2 \pi \mathrm{f}}=\frac{25 \sqrt{2}}{\pi \times 1000} \mathrm{H}$
$=1.125 \times 10^{-2} \mathrm{H}$
