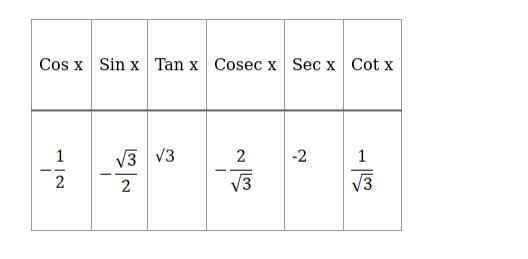
If $\sec x=-2$ and $\pi
Given: $\sec x=-2$
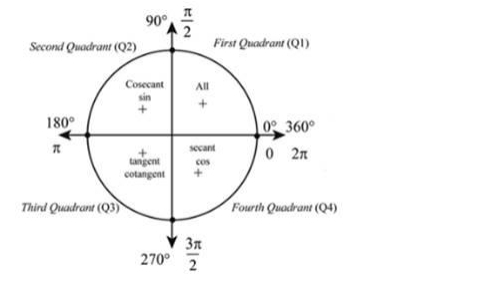
Given that: $\pi So, x lies in IIIrd Quadrant. So, sin and cos will be negative but tan will be positive. Now, we know that $\cos x=\frac{1}{\sec x}$ Putting the values, we get $\cos x=\frac{1}{-2}$ …(i) We know that, $\cos ^{2} x+\sin ^{2} x=1$ Putting the values, we get $\left(-\frac{1}{2}\right)^{2}+\sin ^{2} x=1$ [Given] $\Rightarrow \frac{1}{4}+\sin ^{2} x=1$ $\Rightarrow \sin ^{2} x=1-\frac{1}{4}$ $\Rightarrow \sin ^{2} x=\frac{4-1}{4}$ $\Rightarrow \sin ^{2} x=\frac{3}{4}$ $\Rightarrow \sin x=\sqrt{\frac{3}{4}}$ $\Rightarrow \sin x=\pm \frac{\sqrt{3}}{2}$ Since, $x$ in IIIrd quadrant and $\sin x$ is negative in IIIrd quadrant $\therefore \sin x=-\frac{\sqrt{3}}{2}$ Now, $\tan x=\frac{\sin x}{\cos x}$ Putting the values, we get $\tan x=\frac{-\frac{\sqrt{3}}{2}}{-\frac{1}{2}}$ $=-\frac{\sqrt{3}}{2} \times(-2)$ $=\sqrt{3}$ Now, $\operatorname{cosec} x=\frac{1}{\sin x}$ Putting the values, we get $\operatorname{cosec} x=\frac{1}{-\frac{\sqrt{3}}{2}}$ $=-\frac{2}{\sqrt{3}}$ Now $\cot x=\frac{1}{\tan x}$ Putting the values, we get $\cot x=\frac{1}{\sqrt{3}}$ Hence, the values of other trigonometric Functions are: