Solve this

Let us first draw a right $\triangle \mathrm{ABC}$, right angled at $\mathrm{B}$ and $\angle C=\theta$.

Now, we know that $\tan \theta=\frac{\text { Perpendicular }}{\text { Base }}=\frac{A B}{B C}=\frac{15}{8}$.

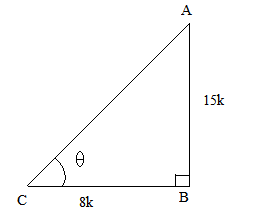
So, if BC = 8k, then AB = 15k, where k is a positive number.
Now, using Pythagoras theorem, we have:
AC2 = AB2 + BC2 = (15k)2 + (8k)2
⇒ AC2 = 225k2 + 64k2 = 289k2
⇒ AC = 17k

Now, finding the other T-ratios using their definitions, we get:

$\sin \theta=\frac{A B}{A C}=\frac{15 k}{17 k}=\frac{15}{17}$

$\cos \theta=\frac{B C}{A C}=\frac{8 k}{17 k}=\frac{8}{17}$

$\therefore \cot \theta=\frac{1}{\tan \theta}=\frac{8}{15}, \operatorname{cosec} \theta=\frac{1}{\sin \theta}=\frac{17}{15}$ and $\sec \theta=\frac{1}{\cos \theta}=\frac{17}{8}$