Question: $\frac{x-1}{x+3}>2$
Solution:
We have, $\frac{x-1}{x+3}>2$
$\Rightarrow \frac{x-1}{x+3}-2>0$
$\Rightarrow \frac{x-1-2(x+3)}{x+3}>0$
$\Rightarrow \frac{x-1-2 x-6}{x+3}>0$
$\Rightarrow \frac{-x-7}{x+3}>0$
$\Rightarrow \frac{x+7}{x+3}<0$
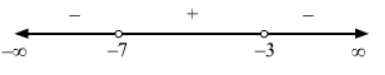
$\therefore x \in(-7,-3)$