Question:
A small particle travelling with a velocity $v$ collides elastically with a spherical body of equal mass and of radius $r$ initially kept at rest. The center of this spherical body is located a distance $(\rho [Hint:- The force acts along the normal to the sphere through the contact. Treat the collision as onedimensional for this direction. In the tangential direction no force acts and velocities do not change.]
Solution:
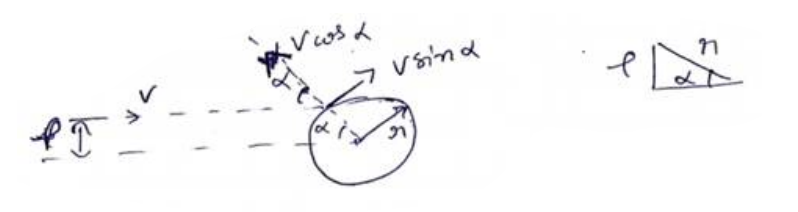
$V_{s p h e r e}=v \cos \alpha=\frac{v}{r} \sqrt{r^{2}-\rho^{2}}$
$V_{\text {particle }}$
\{after collision\}
$=v \sin \alpha=\frac{v p}{r}$
