Question:
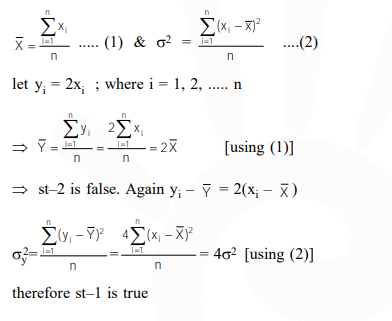
Let $\mathrm{x}_{1}, \mathrm{x}_{2}, \ldots ., \mathrm{x}_{\mathrm{n}}$ be $\mathrm{n}$ observations, and let $\overline{\mathrm{x}}$ be their arithmetic mean and $\sigma^{2}$ be their variance.
Statement-1 : Variance of $2 \mathrm{x}_{1}, 2 \mathrm{x}_{2}, \ldots, 2 \mathrm{x}_{\mathrm{n}}$ is $4 \sigma^{2}$.
Statement-2 : Arithmetic mean of $2 x_{1}, 2 x_{2}, \ldots ., 2 x_{n}$ is $4 \bar{x}$.
Correct Option: 1
Solution: