Question:
Solve each of the following in equations and represent the solution set on the number line.
$6 x \leq 25$, where (i) $x \in N$, (ii) $x \in Z$.
Solution:
(i) $6 x \leq 25, x \in N$
Dividing both the sides by 6 in the above equation,
$\frac{6 x}{6} \leq \frac{25}{6}$
$x \leq \frac{25}{6}$
x ≤ 4.166
Since x is a natural number, therefore the value of x can be less than or equal to 4
Therefore, x = {1,2,3,4}
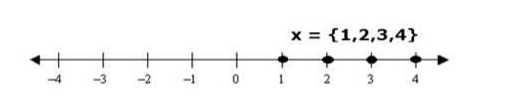
(ii) $6 x \leq 25, x \in Z$
Dividing both the sides by 6 in the above equation,
$\frac{6 x}{6} \leq \frac{25}{6}$
$x \leq \frac{25}{6}$
x ≤ 4.166
Since x is an integer so the possible values of x can be:
$x=\{\ldots,-3,-2,-1,0,1,2,3,4\}$

