Show that the quadrilateral formed by joining the midpoints of the pairs of adjacent sides of a rhombus is a rectangle.

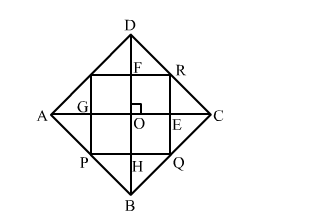
Let ABCD be a rhombus and P, Q, R and S be the midpoints of AB, BC, CD and DA, respectively. 
Join the diagonals, AC and BD.
In ∆ ABC, we have:

$P Q \| A C$ and $P Q=\frac{1}{2} A C$    [By midpoint theorem]

Again, in ∆DAC, the points S and R are the midpoints of AD and DC, respectively.

$\therefore S R \| A C$ and $S R=\frac{1}{2} A C$  [By midpoint theorem]

Now, PQ ∣∣ AC and SR ∣∣ AC  ⇒ PQ ∣∣ SR

Also, PQ = SR     [Each equal to $\frac{1}{2} A C$ ] (i)

So, PQRS is a parallelogram.
We know that the diagonals of a rhombus bisect each other at right angles.
∴ ∠EOF = 90o
​Now, RQ∣∣ DB
 ⇒RE ∣∣ FO
Also, SR∣∣ AC 
⇒FR ∣∣ OE
∴ OERF is a parallelogram.
So, ∠FRE = ∠EOF = 90o​         (Opposite angles are equal)
Thus, PQRS is a parallelogram with ∠R = 90o.
 ∴​ PQRS is a rectangle.