Show that

Solution:

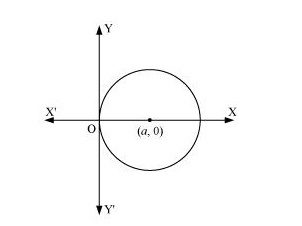
The centre of the circle touching the y-axis at origin lies on the x-axis.

Let (a, 0) be the centre of the circle.

Since it touches the y-axis at origin, its radius is a.

Now, the equation of the circle with centre (a, 0) and radius (a) is

$(x-a)^{2}+y^{2}=a^{2}$

$\Rightarrow x^{2}+y^{2}=2 a x$                 ...(1)

Differentiating equation (1) with respect to x, we get:

$2 x+2 y y^{\prime}=2 a$

$\Rightarrow x+y y^{\prime}=a$

Now, on substituting the value of a in equation (1), we get:

$x^{2}+y^{2}=2\left(x+y y^{\prime}\right) x$

$\Rightarrow x^{2}+y^{2}=2 x^{2}+2 x y y^{\prime}$

$\Rightarrow 2 x y y^{\prime}+x^{2}=y^{2}$\

This is the required differential equation.