Represent 3.5−−−√, 9.4−−−√, 10.5−−−−√ on the real number line.

We are asked to represent the real numbers $\sqrt{3.5}, \sqrt{9.4}$ and $\sqrt{10.5}$ on the real number line

We will follow a certain algorithm to represent these numbers on real number line

(a) $\sqrt{3.5}$

We will take A as reference point to measure the distance

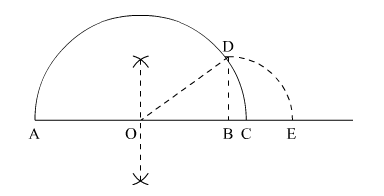
(1) Draw a sufficiently large line and mark a point A on it

(2) Take a point B on the line such that 

(3) Mark a point C on the line such that 

(4) Find mid point of AB and let it be O

(5) Take O as center and OC as radius and draw a semi circle. Draw a perpendicular BD which cuts the semi circle at D

(6) Take B as the center and BD as radius, draw an arc which cuts the horizontal line at E 

(7) Point $E$ is the representation of $\sqrt{3.5}$

(b) $\sqrt{9.4}$

We will take A as reference point to measure the distance. We will follow the same figure in the part (a) 

(1) Draw a sufficiently large line and mark a point A on it

(2) Take a point B on the line such that 

(3) Mark a point C on the line such that 

(4) Find mid point of AB and let it be O

(5) Take O as center and OC as radius and draw a semi circle. Draw a perpendicular BC which cuts the semi circle at D

(6) Take B as the center and BD as radius, draw an arc which cuts the horizontal line at E 

(7) Point $E$ is the representation of $\sqrt{9.4}$

(c) $\sqrt{10.5}$

We will take A as reference point to measure the distance. We will follow the same figure in the part (a) 

(1) Draw a sufficiently large line and mark a point A on 

(2) Take a point B on the line such that 

(3) Mark a point C on the line such that 

(4) Find mid point of AB and let it be O

(5) Take O as center and OC as radius and draw a semi circle. Draw a perpendicular BC which cuts the semi circle at D

(6) Take B as the center and BD as radius, draw an arc which cuts the horizontal line at E 

(7) Point $E$ is the representation of $\sqrt{10.5}