Question:
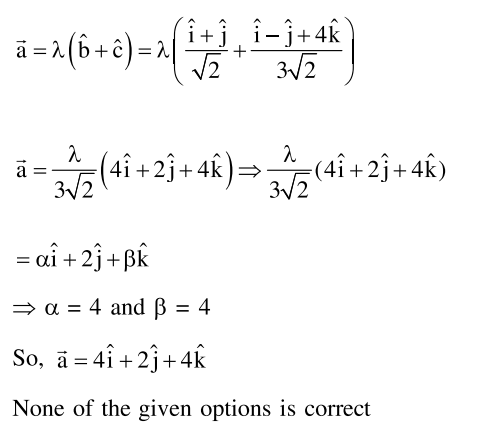
A vector $\vec{a}=\alpha \hat{i}+2 \hat{j}+\beta \hat{k}(\alpha, \beta \in R)$ lies in the plane of the vectros $\vec{b}=\hat{i}+\hat{j}$ and $\vec{c}=\hat{i}-\hat{j}+4 \hat{k}$.
If $\vec{a}$ bisects the angle between $\vec{b}$ and $\vec{c}$, then:
Correct Option: , 4
Solution: