Prove that the ratio of the areas of two similar triangles

Here we have to prove that the ratio of the areas of two similar triangles is equal to the ratio of squares of their corresponding sides.

We have two triangles andin which 

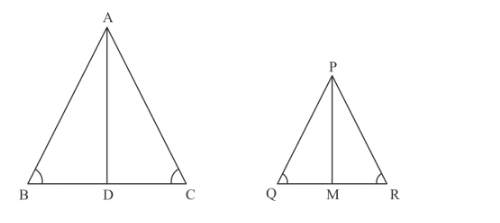
In the given figure AD is perpendicular to BC and PM is perpendicular to QR

The areas of triangle ABC and triangle PQR are given by

Area $(\triangle \mathrm{ABC})=\frac{1}{2} \times$ base $\times$ hight

$=\frac{1}{2} \mathrm{BC} \cdot \mathrm{AD}$

Area $(\Delta \mathrm{PQR})=\frac{1}{2} \times$ base $\times$ hight

$=\frac{1}{2} \mathrm{QR} . \mathrm{PM}$

$\frac{\text { Area }(\mathrm{ABC})}{\text { Area }(\mathrm{PQR})}=\frac{\frac{1}{2} \mathrm{BC} . \mathrm{AD}}{\frac{1}{2} \mathrm{QR} . \mathrm{PM}}$

$\frac{\text { Area }(\mathrm{ABC})}{\text { Area }(\mathrm{PQR})}=\frac{\frac{1}{2} \mathrm{BC} . \mathrm{AD}}{\frac{1}{2} \mathrm{QR} . \mathrm{PM}}$

$=\left(\frac{\mathrm{AD}}{\mathrm{PM}}\right)\left(\frac{\mathrm{BC}}{\mathrm{QR}}\right) \ldots \ldots$(1)

Since are same, therefore

$\sin \mathrm{B}=\frac{\mathrm{AD}}{\mathrm{AB}}$

$\sin Q=\frac{P M}{P Q}$

$\Rightarrow \frac{\mathrm{AD}}{\mathrm{AB}}=\frac{\mathrm{PM}}{\mathrm{PQ}}$

$\Rightarrow \frac{\mathrm{AD}}{\mathrm{PM}}=\frac{\mathrm{AB}}{\mathrm{PQ}}$(2)

By applying the property of similar triangles, we have

$\frac{\mathrm{AB}}{\mathrm{PQ}}=\frac{\mathrm{BC}}{\mathrm{QR}}=\frac{\mathrm{AC}}{\mathrm{PR}}$......(3)

From (2) and (3), we get

$\frac{\mathrm{AD}}{\mathrm{PM}}=\frac{\mathrm{BC}}{\mathrm{QR}}$...(4)

From (1) and (4), we have

$\frac{\text { Area }(\mathrm{ABC})}{\text { Area }(\mathrm{PQR})}=\left(\frac{\mathrm{AD}}{\mathrm{PM}}\right)\left(\frac{\mathrm{BC}}{\mathrm{QR}}\right)$

$=\left(\frac{\mathrm{BC}}{\mathrm{QR}}\right)\left(\frac{\mathrm{BC}}{\mathrm{QR}}\right)$

$=\left(\frac{B C}{Q R}\right)^{2}$

$=\frac{B C^{2}}{Q R^{2}}$

Hence $\frac{\text { Area }(\mathrm{ABC})}{\text { Area }(\mathrm{PQR})}=\frac{\mathrm{BC}^{2}}{\mathrm{QR}^{2}}$