PQ is a tangent drawn from a point P to a circle with centre O and

Let us first put the given data in the form of a diagram.

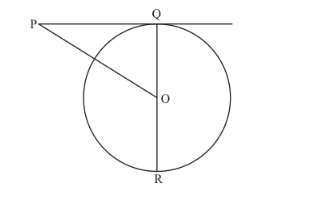
Given data is as follows:

QOR is the diameter.

$\angle P O R=120^{\circ}$

We have to find $\angle O P Q$.

Since QOR is the diameter of the circle, it is a straight line. Therefore,

$\angle Q O R=180^{\circ}$

That is,

$\angle P O R+\angle P O Q=180^{\circ}$

But $\angle P O R=120^{\circ}$. Therefore,

$120^{\circ}+\angle P O Q=180^{\circ}$

$\angle P O Q=60^{\circ}$

Now consider $\triangle P O Q$. We have

$\angle O P Q+\angle P O Q+\angle P Q O=180^{\circ}$ (Sum of all angles of a triangle will be $180^{\circ}$ )

But,

$\angle P Q O=90^{-}$(Since radius will be perpendicular to the tangent at the point of contact)

Therefore,

$\angle O P Q+60^{\circ}+90^{\circ}=180^{\circ}$

$\angle O P Q=30^{\circ}$

Therefore, the correct answer to this question is option (c).