P, Q, R, S are respectively the midpoints of the sides AB, BC, CD and DA of || gm ABCD.

Solution:

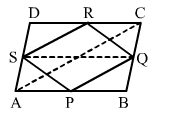
Given:  ABCD is a parallelogram and P, Q, R and S are the midpoints of sides AB, BC, CD and DA, respectively.

To prove: ar(parallelogram $P Q R S)=\frac{1}{2} \times$ ar (parallelogram $A B C D$ )

Proof: 

In $\triangle A B C, P Q \| A C$ and $P Q=\frac{1}{2} \times A C$       [ By midpoint theorem] 

Again, in ∆DAC, the points S and R are the mid points of AD and DC, respectively.

$\therefore \mathrm{SR} \| A C$ and $\mathrm{SR}=\frac{1}{2} \times A C$     [ By midpoint theorem] 

Now, PQ || AC and SR || AC  
⇒ ​PQ || SR

Also, $P Q=S R=\frac{1}{2} \times A C$

∴ PQ || SR and PQ = SR
Hence, PQRS is a parallelogram.

Now, ar(parallelogram​ PQRS) = ar(∆PSQ) + ar(∆SRQ)                       ...(i)
also, ar(parallelogram ABCD) = ar(parallelogram ABQS) + ar(parallelogram SQCD)            ...(ii)

∆PSQ and parallelogram ABQS are on the same base and between the same parallel lines.

So, $\operatorname{ar}(\triangle P S Q)=\frac{1}{2} \times \operatorname{ar}($ parallelogram $A B Q S)$        ...(iii)

Similarly, ∆SRQ and parallelogram SQCD are on the same base and between the same parallel lines.

So, $\operatorname{ar}(\Delta S R Q)=\frac{1}{2} \times \operatorname{ar}($ parallelogram $S Q C D)$     ..(iv)

Putting the values from (iii) and (iv) in (i), we get:

$\operatorname{ar}($ parallelogram $P Q R S)=\frac{1}{2} \times \operatorname{ar}($ parallelogram $A B Q S)+\frac{1}{2} \times \operatorname{ar}($ parallelogram $S Q C D)$

From (ii), we get:

$\operatorname{ar}($ parallelogram $P Q R S)=\frac{1}{2} \times \operatorname{ar}($ parallelogram $A B C D)$