One of the reactions that takes place in producing steel from iron ore is the reduction of iron
One of the reactions that takes place in producing steel from iron ore is the reduction of iron (II) oxide by carbon monoxide to give iron metal and CO2.
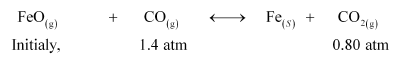
$\mathrm{FeO}(\mathrm{s})+\mathrm{CO}(\mathrm{g}) \longleftrightarrow \mathrm{Fe}(\mathrm{s})+\mathrm{CO}_{2}(\mathrm{~g}) ; \mathrm{K}_{p}=0.265$ at $1050 \mathrm{~K}$
What are the equilibrium partial pressures of $\mathrm{CO}$ and $\mathrm{CO}_{2}$ at $1050 \mathrm{~K}$ if the initial partial pressures are: $p_{\mathrm{CO}}=1.4 \mathrm{~atm}$ and $p_{\mathrm{CO}_{2}}=0.80$ atm?
For the given reaction,
$Q_{\mathrm{p}}=\frac{p_{\mathrm{CO}_{2}}}{p_{\mathrm{CO}}}$
$=\frac{0.80}{1.4}$
$=0.571$
It is given that $K_{\mathrm{p}}=0.265$.
Since $Q_{\mathrm{p}}>K_{\mathrm{p}}$, the reaction will proceed in the backward direction.
Therefore, we can say that the pressure of $\mathrm{CO}$ will increase while the pressure of $\mathrm{CO}_{2}$ will decrease.
Now, let the increase in pressure of $\mathrm{CO}=$ decrease in pressure of $\mathrm{CO}_{2}$ be $p$.
Then, we can write,
$K_{\mathrm{P}}=\frac{p_{\mathrm{CO}_{2}}}{p_{\mathrm{CO}}}$
$\Rightarrow 0.265=\frac{0.80-p}{1.4+p}$
$\Rightarrow 0.371+0.265 p=0.80-p$
$\Rightarrow 1.265 p=0.429$
$\Rightarrow p=0.339 \mathrm{~atm}$
Therefore, equilibrium partial of $\mathrm{CO}_{2}, p_{\mathrm{CO}_{2}}=0.80-0.339=0.461 \mathrm{~atm}$.
And, equilibrium partial pressure of $\mathrm{CO}, p_{\mathrm{CO}}=1.4+0.339=1.739 \mathrm{~atm}$.