A $10 \mu \mathrm{F}$ capacitor is fully charged to a potential difference of $50 \mathrm{~V}$. After removing the source voltage it is connected to an uncharged capacitor in parallel. Now the potential difference across them becomes $20 \mathrm{~V}$. The capacitance of the second capacitor is:
Correct Option: 2,
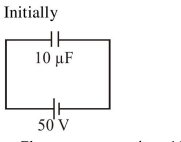
- Charge on capacitor $10 \mu \mathrm{F}$
$\mathrm{Q}=\mathrm{CV}=(10 \mu \mathrm{F})(50 \mathrm{~V})$
$\mathrm{Q}=500 \mu \mathrm{C}$

- Final Charge on $10 \mu \mathrm{F}$ capacitor
$\mathrm{Q}=\mathrm{CV}=(10 \mu \mathrm{F})(20 \mathrm{~V})$
$\mathrm{Q}=200 \mu \mathrm{C}$
- From charge conservation,
Charge on unknown capacitor
$C=500 \mu C-200 \mu C=300 \mu C$
$\Rightarrow$ Capacitance $(\mathrm{C})=\frac{\mathrm{Q}}{\mathrm{V}}=\frac{300 \mu \mathrm{C}}{20 \mathrm{~V}}=15 \mu \mathrm{F}$
