Question:
Lines are drawn parallel to the line $4 x-3 y+2=0$,
at a distance $\frac{3}{5}$ from the origin.
Then which one of the following points lies on any of these lines?
Correct Option: 1
Solution:
Required line is $4 x-3 y+\lambda=0$
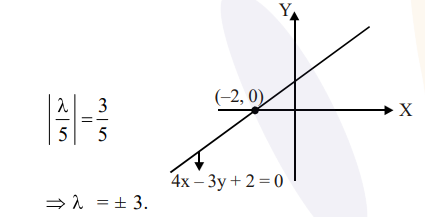
So, required equation of line is $4 x-3 y+3=0$ and $4 x-3 y-3=0$
(1) $4\left(-\frac{1}{4}\right)-3\left(\frac{2}{3}\right)+3=0$
Click here to get exam-ready with eSaral
For making your preparation journey smoother of JEE, NEET and Class 8 to 10, grab our app now.