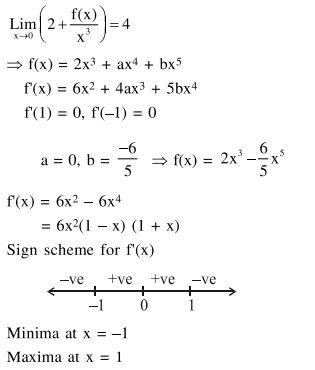
Question: Let $f(x)$ be a polynomial of degree 5 such that $x=\pm 1$ are its critical points. If
$\lim _{x \rightarrow 0}\left(2+\frac{f(x)}{x^{3}}\right)=4$, then which one of the following is not true?
$f$ is an odd function
$x=1$ is a point of minima and $x=-1$ is a point of maxima of $f$.
$x=1$ is a point of maxima and $x=-1$ is a point of minimum of $f$.
$f(1)-4 f(-1)=4$
Correct Option: , 2
Solution: