Question.
Joseph jogs from one end A to the other end B of a straight 300 m road in 2 minutes 50 seconds and then turns around and jogs 100 m back to point C in another 1 minute. What are Joseph's average speeds and velocities in jogging (a) from A to B and (b) from A to C ?
Joseph jogs from one end A to the other end B of a straight 300 m road in 2 minutes 50 seconds and then turns around and jogs 100 m back to point C in another 1 minute. What are Joseph's average speeds and velocities in jogging (a) from A to B and (b) from A to C ?
Solution:
The required figure is as shown
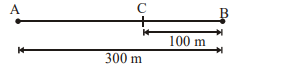
(a) Distance covered = 300 m
Time taken $=2 \mathrm{~min}$ and $50 \mathrm{~s}=170 \mathrm{~s}$
Now average speed from $A$ to $B$ is given by
$\mathrm{V}_{\text {av }}=\frac{\text { distance covered }}{\text { time }}=\frac{300}{170}=1.76 \mathrm{~ms}^{-1}$
Now average velocity from A to B is given by
$V_{\text {av }}=\frac{\text { displacement }}{\text { time }}=\frac{300}{170}=1.76 \mathrm{~ms}^{-1}$
(b) When Joseph turns around from B to C towards
west, then
Distance covered $=300+100=400 \mathrm{~m}$
Time taken $=170+60=230 \mathrm{~s}$
Therefore, average speed from $\mathrm{A}$ to $\mathrm{C}$ is
$\mathrm{V}_{\mathrm{av}}=\frac{\text { distance covered }}{\text { time }}=\frac{400}{230}=1.74 \mathrm{~ms}^{-1}$
Now displacement from $\mathrm{A}$ to $\mathrm{C}=200 \mathrm{~m}$
Therefore, average velocity from $\mathrm{A}$ to $\mathrm{C}$ is
$\mathrm{V}_{\mathrm{av}}=\frac{\text { displacement }}{\text { time }}=\frac{200}{230}=0.869 \mathrm{~ms}^{-1}$
The required figure is as shown

(a) Distance covered = 300 m
Time taken $=2 \mathrm{~min}$ and $50 \mathrm{~s}=170 \mathrm{~s}$
Now average speed from $A$ to $B$ is given by
$\mathrm{V}_{\text {av }}=\frac{\text { distance covered }}{\text { time }}=\frac{300}{170}=1.76 \mathrm{~ms}^{-1}$
Now average velocity from A to B is given by
$V_{\text {av }}=\frac{\text { displacement }}{\text { time }}=\frac{300}{170}=1.76 \mathrm{~ms}^{-1}$
(b) When Joseph turns around from B to C towards
west, then
Distance covered $=300+100=400 \mathrm{~m}$
Time taken $=170+60=230 \mathrm{~s}$
Therefore, average speed from $\mathrm{A}$ to $\mathrm{C}$ is
$\mathrm{V}_{\mathrm{av}}=\frac{\text { distance covered }}{\text { time }}=\frac{400}{230}=1.74 \mathrm{~ms}^{-1}$
Now displacement from $\mathrm{A}$ to $\mathrm{C}=200 \mathrm{~m}$
Therefore, average velocity from $\mathrm{A}$ to $\mathrm{C}$ is
$\mathrm{V}_{\mathrm{av}}=\frac{\text { displacement }}{\text { time }}=\frac{200}{230}=0.869 \mathrm{~ms}^{-1}$
