Question:
In the given figure, O is the centre of the circle and BCD is tangent to it at C. Prove that ∠BAC + ∠ACD = 90°.
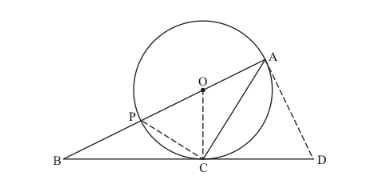
Solution:
In the given figure, let us join D an A.
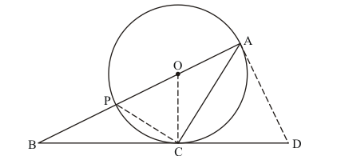
Consider 
. We have,
OC = OA (Radii of the same circle)
We know that angles opposite to equal sides of a triangle will be equal. Therefore,
…… (1)
It is clear from the figure that
Now from (1)
Now as BD is tangent therefore 
Therefore 
From the figure we can see that 
Thus we have proved.