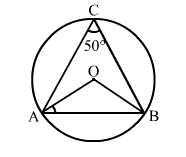
In the given figure, O is the centre of the circle.

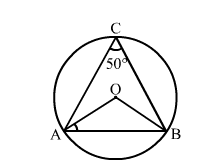
We know that the angle subtended by an arc of a circle at the centre is double the angle subtended by the arc at any point on the circumference.
∠AOB = 2∠ACB

 = 2 × 50°      [Given]

∠AOB = 100°       ...(i)
Let us consider the triangle ΔOAB.
OA = OB (Radii of a circle)
Thus, ∠OAB = ∠OBA 
In ΔOAB, we have:
∠AOB + ∠OAB + ∠OBA = 180°
⇒ 100° + ∠OAB + ∠OAB = 180°
⇒ 100° + 2∠OAB = 180°
⇒ 2∠OAB = 180° – 100° = 80°
⇒ ∠OAB = 40°
Hence, ∠OAB = 40°