Question:
In the given figure, O is the centre of a circle, BOA is its diameter and the tangent at the point P meets BA extended at T. If ∠PBO = 30∘ then ∠PTA = ?
(a) 60∘
(b) 30∘
(c) 15∘
(d) 45∘
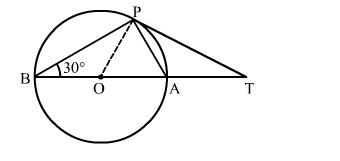
Solution:
We know that a chord passing through the centre is the diameter of the circle.
∵∠BPA = 90∘ (Angle in a semi circle is 90∘)
By using alternate segment theorem
We have ∠APT = ∠ABP = 30∘
Now, In △ABP
∠PBA + ∠BPA + ∠BAP = 1800 [Angle sum property of a triangle]
⇒ 30∘ + 900 + ∠BAP = 180∘
⇒ ∠BAP = 60∘
Now, ∠BAP = ∠APT + ∠PTA
⇒ 60∘ = 30∘ + ∠PTA
⇒ ∠PTA = 30∘
Hence, the correct answer is option (b).
