In the given figure, JKLM is a square with sides of length 6 units.

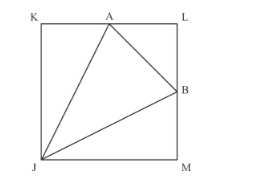
Given: JKLM is a square with sides of length 6units. Points A and B are the midpoints of sides KL and ML respectively. If a point is selected at random from the interior of the square

To find: Probability that the point will be chosen from the interior of ΔJAB.

We the following figure

Area of square JLKM is equal to

$=6^{2}$

$=36 \mathrm{sq}$ units

Now we have

$\operatorname{ar}(\Delta \mathrm{KAJ})=\frac{1}{2} \times \mathrm{AK} \times \mathrm{KJ}$

$=\frac{1}{2} \times 3 \times 6$

$=9$ units $^{2}$

$\operatorname{ar}(\Delta \mathrm{JMB})=\frac{1}{2} \times \mathrm{JM} \times \mathrm{BM}$

$=\frac{1}{2} \times 6 \times 3$

$=9$ units $^{2}$

$\operatorname{ar}(\Delta \mathrm{ALB})=\frac{1}{2} \times \mathrm{AL} \times \mathrm{BL}$

$=\frac{1}{2} \times 3 \times 3$

$=\frac{9}{2}$ units $^{2}$

Now area of the triangle AJB

$\operatorname{ar}(\Delta \mathrm{AJB})=36-9-9-\frac{9}{2}$

$=\frac{27}{2}$ units $^{2}$

We know that Probability

$=\frac{\text { Number of favourable event }}{\text { Total number of event }}$

$=\frac{\frac{27}{2}}{36}$

$=\frac{27}{2 \times 36}$

$=\frac{3}{8}$

Hence the Probability that the point will be chosen from the interior of $\triangle \mathrm{AJB}$ is $\frac{3}{8}$