In the given figure, if AD, AE and BC are tangents to the circle at D, E and F respectively, Then,
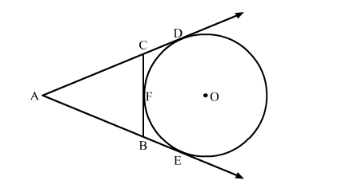
(a) AD = AB + BC + CA
(b) 2AD = AB + BC + CA
(c) 3AD = AB + BC + CA
(d) 4AD = AB + BC + CA
In the given problem, the Right Hand Side of all the options is same, that is,
AB + BC + CA
So, we shall find out AB + BC + CA and check which of the options has the Left Hand Side value which we will arrive at.
By looking at the figure, we can write,
AB + BC + CA = AB + BF + FC + CD
We know that tangents drawn from an external point will be equal in length. Therefore,
BF = BE
FC= CD
Now we have,
AB + BC + CA = AB + BE + CD + CA
AB + BC + CD = (AB + BE) + (CD + CA)
By looking at the figure, we write the above equation as,
AB + BC + CD = AE + AD
Since tangents drawn from an external point will be equal,
AE = AD
Therefore,
AB + BC + CD = AD + AD
AB + BC + CD = 2AD
Therefore option (b) is the correct answer.
