In the given figure, AP is a tangent to the circle with centre O such that OP = 4 cm and ∠OPA = 30°. Then, AP =
In the given figure, AP is a tangent to the circle with centre O such that OP = 4 cm and ∠OPA = 30°. Then,
AP =
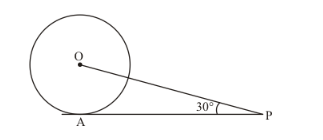
(a) $2 \sqrt{2} \mathrm{~cm}$
(b) $2 \mathrm{~cm}$
(c) $2 \sqrt{3} \mathrm{~cm}$
(d) $3 \sqrt{2} \mathrm{~cm}$
In the given figure,
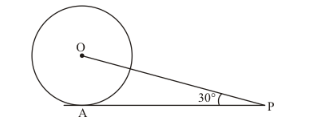
if we join O and A, the line OA will be perpendicular to AP. This is because the radius of a circle will always be perpendicular to the tangent at the point of contact. Therefore, ![]() is a right triangle.
is a right triangle.
We know that,
$\cos \theta=\frac{\text { Adjacent side }}{\text { Hypotenuse }}$
$\cos \angle A P O=\frac{A P}{O P} \cdots \cdots$(1)
We know that, $\cos 30^{\circ}=\frac{\sqrt{3}}{2}$
$\cos \angle A P O=\frac{\sqrt{3}}{2}$.......(2)
From equations (1) and (2), we have,
$\frac{A P}{O P}=\frac{\sqrt{3}}{2}$
$A P=\frac{\sqrt{3}}{2} O P$
$A P=\frac{\sqrt{3}}{2} \times 4$
$A P=2 \sqrt{3}$
Therefore, option (c) is the correct answer.
