In the given figure, a square OABC has been inscribed in the quadrant OPBQ. If OA = 20 cm, then the area of the shaded region is
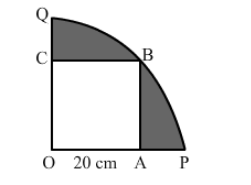
(a) 214 cm2
(b) 228 cm2
(c) 242 cm2
(d) 248 cm2
(b) 228 cm2
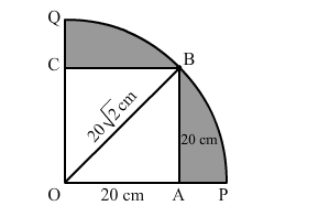
Join OB.
Now, OB is the radius of the circle.
We have :
$O B^{2}=O A^{2}+A B^{2} \quad$ [By Pythagoras' theorem]
$\Rightarrow O B^{2}=\left\{(20)^{2}+(20)^{2}\right\} \mathrm{cm}^{2}$
$\Rightarrow O B^{2}=(400+400) \mathrm{cm}^{2}$
$\Rightarrow O B^{2}=800 \mathrm{~cm}^{2}$
$\Rightarrow O B=20 \sqrt{2} \mathrm{~cm}$
Hence, the radius of the circle is $20 \sqrt{2} \mathrm{~cm}$.
Now,
Area of the shaded region = Area of the quadrant
$=\left|\left(\frac{1}{4} \times 3.14 \times 20 \sqrt{2} \times 20 \sqrt{2}\right)-(20 \times 20)\right| \mathrm{cm}^{2}$
$=\left|\left(\frac{1}{4} \times \frac{314}{100} \times 800\right)-400\right| \mathrm{cm}^{2}$
$=(628-400) \mathrm{cm}^{2}$
$=228 \mathrm{~cm}^{2}$
