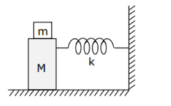
In the given figure, a mass M is attached to a horizontal spring which is fixed on one side to a rigid support.
In the given figure, a mass $M$ is attached to a horizontal spring which is fixed on one side to a rigid support. The spring constant of the spring is $\mathrm{k}$. The mass oscillates on a frictionless surface with time period $T$ and amplitude A. When the mass is in equilibrium position, as shown in the figure, another mass $m$ is gently fixed upon it. The new amplitude of oscillation will be -
Correct Option: 1
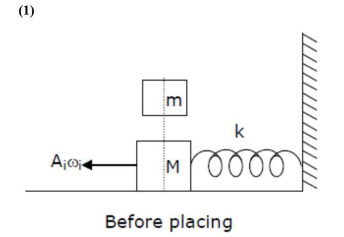
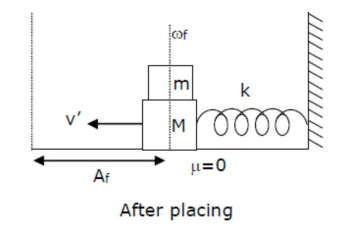
We know that $\omega=\sqrt{\frac{\mathrm{k}}{\mathrm{m}}}$ and $\omega_{\mathrm{i}}=\sqrt{\frac{\mathrm{k}}{\mathrm{M}}} \quad \mathrm{A}_{\mathrm{i}}=\mathrm{A}$
Also, momentum is conserved just before and just after the block of mass $(\mathrm{m})$ is placed because there is no implusive force. So -
$M A_{i} \omega_{i}=(M+m) v^{\prime}$
$v^{\prime}=\frac{M A_{i} \omega_{i}}{(M+m)} \Rightarrow v^{\prime}=A_{f} \omega_{f}$
$\frac{M A \omega_{i}}{(M+m)}=A_{f} \sqrt{\frac{K}{(M+m)}}$
$\Rightarrow \frac{M A}{M+m} \times \sqrt{\frac{K}{M}} \times \sqrt{\frac{M+m}{K}}=A_{f}$
$\Rightarrow A_{f}=A \sqrt{\frac{M}{(M+m)}}$
