In the given figure, a circle is inscribed in a quadrilateral ABCD in which ∠B = 90°. It AD = 23 cm, AB = 29 cm and DS = 5 cm, find the radius r of the circle.
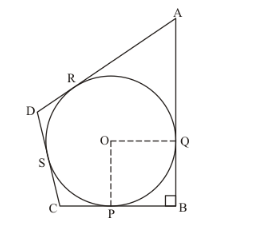
Let us first consider the quadrilateral OPBQ.
It is given that ![]() .
.
Also from the property of tangents we know that the radius of the circle will always be perpendicular to the tangent at the point of contact. Therefore we have,
![]()
We know that sum of all angles of a quadrilateral will always be equal to ![]() . Therefore,
. Therefore,
$\angle B+\angle O P B+\angle O Q B+\angle P O Q=360^{\circ}$
$90^{\circ}+90^{\circ}+90^{\circ}+\angle P O Q=360^{\circ}$
$270^{\circ}+\angle P O Q=360^{\circ}$
$\angle P O Q=90^{\circ}$
Also, in the quadrilateral,
OQ = OP (both are the radii of the same circle)
PB = BQ (from the property of tangents which says that the length of two tangents drawn to a circle from the same external point will be equal)
Since the adjacent sides of the quadrilateral are equal and also since all angles of the quadrilateral are equal to 90°, we can conclude that the quadrilateral OPBQ is a square.
It is given that DS = 5 cm.
From the property of tangents we know that the length of two tangents drawn from the same external point will be equal. Therefore,
DS = DR
DR = 5
It is given that,
AD = 23
DR + RA = 23
5 + RA = 23
RA = 18
Again from the same property of tangents we have,
RA = AQ
We have found out RA = 18. Therefore,
AQ = 18
It is given that AB = 29. That is,
AQ + QB = 29
18 + QB = 29
QB = 11
We have initially proved that OPBQ is a square. QB is one of the sides of the square. Since all sides of the square will be of equal length, we have,
OP = 11
OP is nothing but the radius of the circle.
Thus the radius of the circle is equal to 11 cm.
