In the following figure, the boundary of the shaded region consists

Solution:

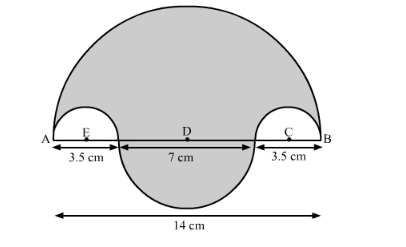
(i) We will first find the length of the boundary.

Length of the boundary  perimeter of semi-circle with diameter AB + boundary of semi-circle with diameter 7 cm

Length of the boundary $=(\pi \times 7+\pi \times 3.5)+\pi \times 1.75+\pi \times 1.75$

$\therefore$ Length of the boundary $=14 \pi$

 

$\therefore$ Length of the boundary $=44$

Therefore, length of the boundary is $44 \mathrm{~cm}$.

Now we will find the area of the shaded region as shown below,

Area of the shaded region = Area of the semi-circle with AB as a diameter − area of the semi-circle with radius AE − area of the semi-circle with radius BC + area of the semi-circle with diameter 7 cm.

$\therefore$ Area of the shaded region $=\frac{\pi \times 7 \times 7}{2}-\frac{\pi \times 1.75 \times 1.75}{2}-\frac{\pi \times 1.75 \times 1.75}{2}+\frac{\pi \times 3.5 \times 3.5}{2}$

Area of the shaded region $=\frac{49 \pi}{2}-3.0625 \pi+\frac{12.25 \pi}{2}$

$\therefore$ Area of the shaded region $=\frac{61.25 \pi}{2}-3.0625 \pi$

$\therefore$ Area of the shaded region $=\frac{61.25 \pi-6.125 \pi}{2}$

$\therefore$ Area of the shaded region $=55.125 \times \frac{22}{7} \times \frac{1}{2}$

$\therefore$ Area of the shaded region $=7.875 \times 11$

 

$\therefore$ Area of the shaded region $=86.625$

Therefore, area of the shaded region is $86.625 \mathrm{~cm}^{2}$.